Calculus limit formula
An example is a garage door with two limit switches one for UP labelled SW_U and one for DOWN labelled SW_D and whatever else is in the doors circuitry. The laws of physics are invariant that is identical in all inertial frames of reference that is frames of reference with no acceleration.

Integration Calculus Maths Algebra Formulas Math Formulas Studying Math
In this section we are going to look at the derivatives of the inverse trig functions.

. In this article we are going to discuss the formula and proof for the LHospitals rule along with examples. We have two formulas to evaluate a definite integral as mentioned below. Arc length is the distance between two points along a section of a curve.
B is the period so you can elongate or shorten the period by changing that constant. Maxwells equations can be formulated with possibly time-dependent surfaces and volumes by using the differential version and using Gauss and Stokes formula appropriately. Mathematics from Ancient Greek μάθημα.
Latinized as Alhazen c. In fact many infinite limits are actually quite easy to work out when we figure out which way it is going like this. In physics the special theory of relativity or special relativity for short is a scientific theory regarding the relationship between space and timeIn Albert Einsteins original treatment the theory is based on two postulates.
At present Ive gotten the notestutorials for my Algebra Math 1314 Calculus I Math 2413 Calculus II Math 2414 Calculus III Math 3435 and Differential Equations Math 3301 class online. Again we need to keep in mind that as we rewrite the limit in terms of other limits each new limit must exist for the limit law to be applied. Ive also got a couple of ReviewExtras available as well.
For this simple equation you could stop there and assume that the limit from the right is going to equal the same thing. Explore the entire Calculus curriculum. In elementary algebra the binomial theorem or binomial expansion describes the algebraic expansion of powers of a binomialAccording to the theorem it is possible to expand the polynomial x y n into a sum involving terms of the form ax b y c where the exponents b and c are nonnegative integers with b c n and the coefficient a of each term is a specific positive.
But dont be fooled by the. Putting the stated x-value 1 into the x formula you get. A left-hand limit value will tend to 0 the right-hand limit value to.
The fundamental theorem of calculus is a theorem that links the concept of differentiating a function calculating the gradient with the concept of integrating a function calculating the area under the curve. In essence it is the Newton interpolation formula first published in his Principia Mathematica in 1687. Change of base formula 5.
Inspection of the circuit either the diagram or the actual objects themselvesdoor switches. 21 June 14 November 1716 was a German polymath active as a mathematician philosopher scientist and diplomatHe is one of the most prominent figures in both the history of philosophy and the history of mathematicsHe wrote works on philosophy theology ethics politics law history and philology. The formal calculus of finite differences can be viewed as an alternative to the calculus of infinitesimals.
Definite integral formulas are used to evaluate a definite integral. In calculus the limit of functions is still a kind of maximum. Knowledge study learning is an area of knowledge that includes such topics as numbers arithmetic and number theory formulas and related structures shapes and the spaces in which they are contained and quantities and their changes calculus and analysis.
We have seen two examples one went to 0 the other went to infinity. In Calculus the most important rule is L Hospitals Rule LHôpitals rule. Calculus is divided into two separate categories.
The first part of the theorem sometimes. Calculus found its outstanding success partly because it replaced tedious exhaustion arguments with short routine calculations. Lim x 2 2 x 2 3 x 1 x 3 4 lim x 2 2 x 2 3 x 1 lim x 2 x 3 4 Apply the quotient law making sure that.
The process of finding derivatives of a function is called differentiation in calculus. But there are sometimes. Determining the length of an irregular arc segment by approximating the arc segment as connected straight line segments is also called curve rectificationA rectifiable curve has a finite number of segments in its rectification so the curve has a finite length.
Calculus is a branch of mathematics that deals with the calculations related to continuously changing quantities. And they make use of the fundamental notions of convergence of infinite sequences and infinite series to a well-defined limit. We use the language of calculus to describe graphs of functions.
The first formula is called the definite integral as a limit sum and the second formula is called the fundamental theorem of calculus. Set students up for success in Calculus and beyond. Gottfried Wilhelm von Leibniz 1 July 1646 OS.
In fact the sign comes into the propositional calculus when a formula is to be evaluated. The two operations are inverses of each other apart from a constant value which is dependent on where one starts to compute area. Quotient property of logarithms.
Infinitesimal calculus was developed independently in the late 17th century by Isaac Newton and Gottfried. Two young mathematicians discuss optimization from an abstract point of view. In order to derive the derivatives of inverse trig functions well need the formula from the last section relating the.
Find the differentiation of y x 3 5 x 2 3x 7. 1040 CE derived a formula for the sum of. Derivatives of Inverse Trig Functions.
A derivative is the rate of change of a function with respect to another quantity. Calculus gave mathematicians a set of rules to follow not tied down with long logical justifications1. Find the limit at a.
A is the amplitude. The derivative of a function f at a point x is defined by the limit. Calculus originally called.
Limit math is one of the most important concepts in Calculus. Product property of logarithms 6. Most mathematical activity involves the use of pure.
1 1 So the limit as you approach from the left is 1. If a curve can be parameterized as an. Section 3-7.
Ω displaystyle scriptstyle partial Omega is a surface integral over the boundary surface Ω with the loop indicating the surface is closed. Polynomials derivatives and more. Named after Isaac Newton.
But we might not be aware of vector calculus. The limit of a continuous function at a point is equal to the value of the function at that point. The math limit formula can be defined as the value that a.
We cannot actually get to infinity but in limit language the limit is infinity which is really saying the function is limitless. We know that calculus can be classified into two different types such as differential calculus and integral calculus. One of the main differences in the graphs of the sine and sinusoidal functions is that you can change the amplitude period and other features of the sinusoidal graph by tweaking the constantsFor example.
In this article we are going to discuss the definition of vector calculus formulas applications line integrals the surface integrals in detail. We want to give the answer 2 but cant so instead mathematicians say exactly what is going on by using the special word limit The limit of x 2 1 x1 as x approaches 1 is 2 And it is written in symbols as.

Digital Wish Apps Center Calculus Quick Reference Free Learning Mathematics Math Methods Math Formulas

Calculus I Limits At Infinity Part I Studying Math Calculus Physics And Mathematics

Evaluating Limits 9 Math Formulas Calculus Studying Math

Some Remarkable Limits Gcse Math Mathematics Calculus

Free Calculus Derivatives And Limits Mathematics Worksheets Calculus Maths Algebra Formulas
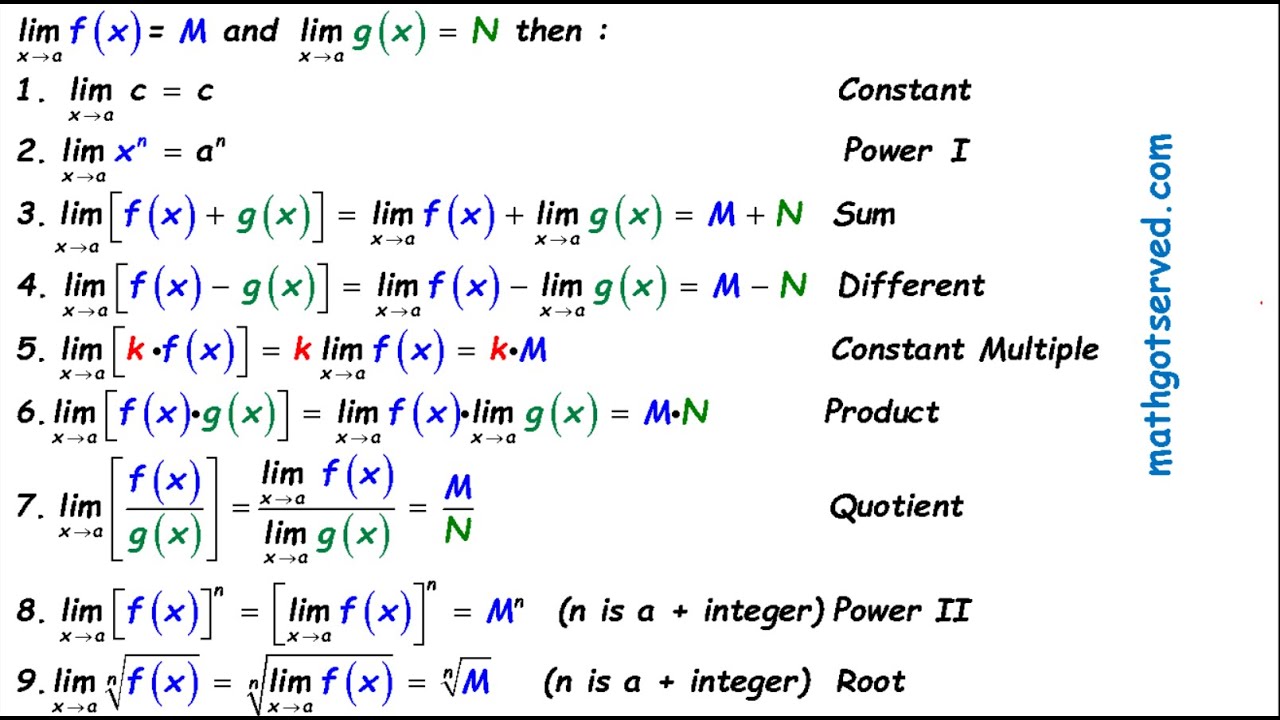
Limit Laws To Evaluate Understand Limits Ap Calculus Ab Bc Exam Mathgots Ap Calculus Ab Ap Calculus Calculus

Calculus I Limits At Infinity Part I Math Methods Online Math Help Calculus

Lthmath Incredibly Useful Take Your Time To Understand Exactly How Differentiation Is Defined Using The Concept Of Studying Math Math Methods Learning Math

Pauls Online Notes Calculus I Limits At Infinity Part I Learning Mathematics Calculus Physics And Mathematics

Multiple Representations And Limit Definition Of The Derivative In Ap Calc Give Me A Sine Calculus Ap Calculus Learning Math

Practical Centre Application Of Differential Calculus Basic Definition Formulas Mathematics Xii Differential Calculus Calculus Mathematics

Google Image Result For Https Www Pioneermathematics Com Formulasimages Imp 20limits Contd 1 Gif Quotient Rule Power Rule Formula

Evaluating Limits A Plus Topper Math Quotes Math Formulas Studying Math

Evaluating Limits A Plus Topper Limits Calculus Studying Math Math Formulas

Which One Doesn T Belong Limits Ap Calculus Ap Calculus Calculus Math Puns

Step 1 N Th Term Test Math Formulas Mathematics Calculus

Derivative Of Logarithmic Function For More Such Calculus Problems Log On To Http Www Assignmenthelp Net Math Assign Math Answers Studying Math Math Methods